报告题目：Determinantal Hypersurfaces, Joint Spectra, and Representations of Coxeter Groups

报告人：Michael Stessin  纽约州立大学阿尔巴尼分校

报告时间：2019年5月28日星期二  上午10:00-11:00     2019年5月29日星期三  上午10:00-11:00

报告地点：创新园大厦 B1410

报告校内联系人：卢玉峰  教 授  联系电话：84708351-8127

报告摘要：This talk is based on joint works with Z. Cuckovic, T. Peebles, A. Tchernev and J. Weyman. Given a tuple  of NXN matrices, determinantal hypersurface 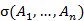 is an algebraic set in the complex projective space  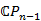given by 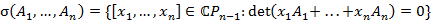. In the infinite dimensional case of operators acting on a Hilbert space, the corresponding set is called the projective joint spectrum and is defined by 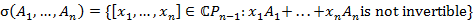. The main topic of the talk is how the geometry of the joint spectrum reveals a mutual behavior of the operators in the tuple. We’ll see that this investigation leads to a characterization of representations of finite Coxeter groups in terms of determinantal hypersurfaces.

报告人简介：Michael Stessin是纽约州立大学阿尔巴尼分校数学与统计系系主任,研究兴趣包括逼近论、算子理论、函数空间理论、多复分析等。在Trans. Amer. Math. Soc., J. Funct. Anal., J. Approx. Theory, J. Anal. Math. 等杂志上发表多篇论文。

437ccm必赢国际首页欢迎您

2019年5月27日